Dimitri Van De Ville

Multidimensional Wavelet Design

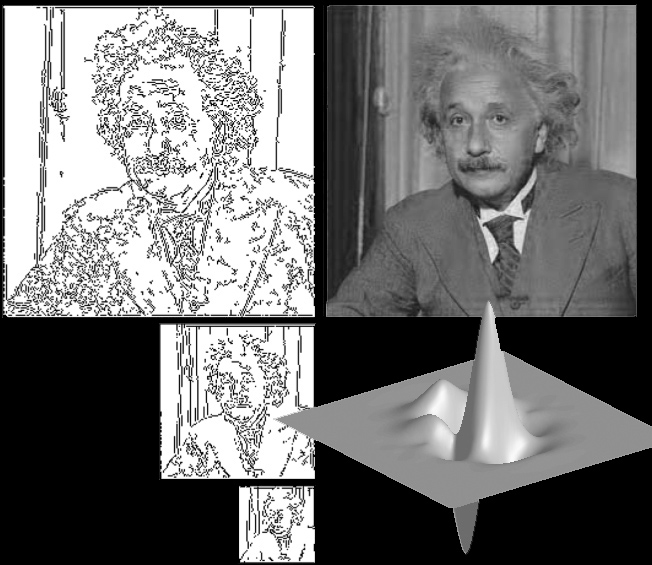Our aim in this work is to tighten the link between wavelets, some classical image-processing operators, and David Marr's theory of early vision. The cornerstone of our approach is a new complex wavelet basis that behaves like a smoothed version of the Gradient-Laplace operator. Starting from first principles, we show that a single-generator wavelet can be defined analytically and that it yields a semi-orthogonal complex basis of L₂(R²), irrespective of the dilation matrix used. We also provide an efficient FFT-based filterbank implementation. We then propose a slightly redundant version of the transform that is nearly translation-invariant and that is optimized for better steerability (Gaussian-like smoothing kernel). We call it the Marr-like wavelet pyramid because it essentially replicates the processing steps in Marr's theory of early vision. We use it to derive a primal wavelet sketch which is a compact description of the image by a multiscale, subsampled edge map. Finally, we provide an efficient iterative algorithm for the reconstruction of an image from its primal wavelet sketch.

This work is in collaboration with Prof. Michael Unser (EPFL) and Dr. Brigitte Forster-Heinlein (TUM).

Analytic Sensing for EEG Source Localization

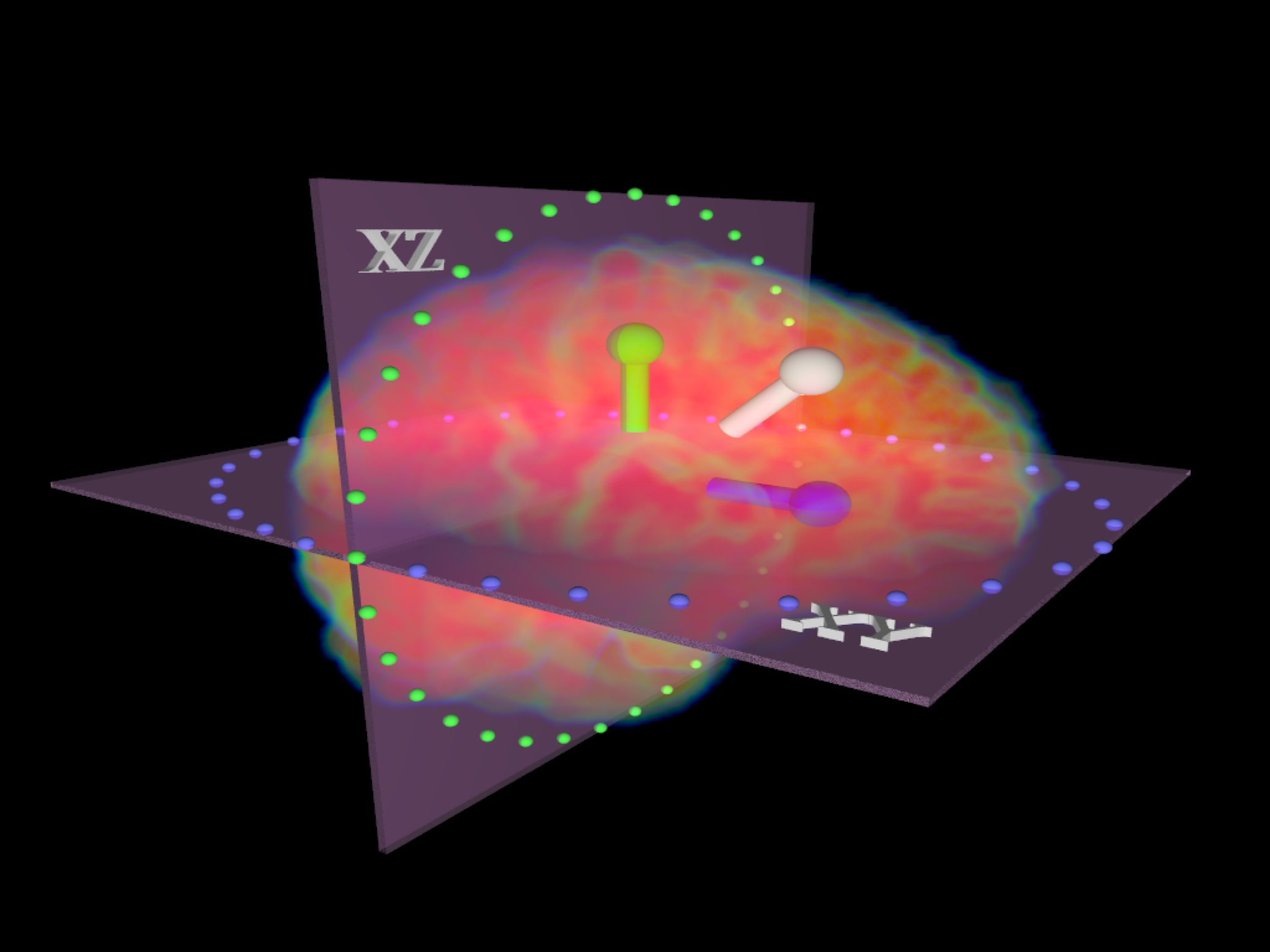We propose a new theoretical framework that leads to a non-iterative technique for EEG source imaging. We designate our method as analytic sensing, since the key contribution is to apply analytic sensors (functions with vanishing Laplacian in some domain) that sense the influence of the source distribution in a specific region.
Our approach can be applied for multi-pole or multi-dipole source models, bringing together several attractive features: (1) the non-linear (dipole positions) and linear (dipolar moments) estimation steps are decoupled; (2) the non-linear estimation is direct (non-iterative) and fast; (3) no forward model is needed, while the scalp surface can be non-spherical; (4) the method can be spatially selective to only incorporate the influence of sources in a desired region-of-interest.

This work is in collaboration with Djano Kandaswamy (PhD student) and Prof. Thierry Blu (Chinese University of Hong Kong), Prof. Christoph Michel (UniGE, EEG Lab), and Dr. Laurent Spinelli (HUG, Functional Brain Mapping Unit).

Resting State FMRI

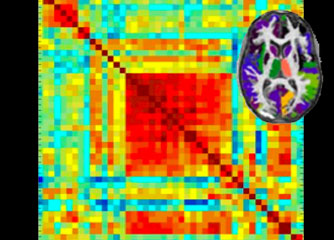Brain functioning during resting state appears to be an enormous source of information to neuroscientists. Some brain networks consistently activate when no task is present and their modulation gives new insights in cognitive aspects of brain functioning. Moreover, efficient and robust detection of resting-state network can become increasingly important as an early-detection biomarker for various pathologies. The image at the right side illustrates a correlation matrix between preselected brain regions for one particular wavelet scale.

This work is in collaboration with Hamdi Eryilmaz (PhD student), and Prof. Patrik Vuilleumier and Dr. Sophie Schwartz (UniGE, LABNIC). Related work using simultaneous EEG-fMRI recordings is done in collaboration with Dr. Juliane Britz (UniGE, EEG Lab).

Laser Doppler Imaging for Intra-Operative Brain Mapping

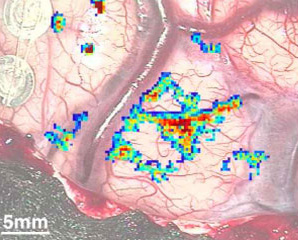The identification and accurate location of centers of brain activity are vital both in neurosurgery and brain research. This study aimed to provide a non-invasive, non-contact, accurate, rapid and user-friendly means of producing functional images intraoperatively. To this end a full field laser Doppler imager was developed and integrated within the surgical microscope and perfusion images of the cortical surface were acquired during awake surgery whilst the patient performed a predetermined task. The regions of brain activity showed a clear signal (10-20 percent with respect to the baseline) related to the stimulation protocol which lead to intraoperative functional brain maps of strong statistical significance and which correlate well with the preoperative fMRI and intraoperative cortical electro-stimulation. These initial results achieved with a prototype device and wavelet based regressor analysis (the hemodynamic response function being derived from MRI applications) demonstrate the feasibility of LDI as an appropriate technique for intraoperative functional brain imaging

This work is in collaboration with Prof. Theo Lasser (EPFL, LOB). Related work on Monte-Carlo simulations is done with Dr. Tiziano Binzoni (UniGE).

Mind reading techniques for FMRI

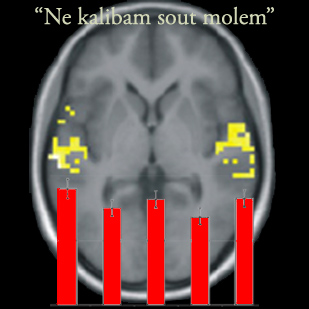Multivariate pattern analysis has become a key approach to perform ``brain reading'' from FMRI acquisitions; i.e., predict the subject's activity from the data. We showed that it is possible to correctly decode the emotion from pseudowords ("Ne kalibam sout molem") spoken in five prosodic categories (anger, sadness, neutral, relief, joy) on the basis of the spatial response pattern within the auditory cortex only. These results demonstrate for the first time that emotional information is represented by distinct spatial patterns that can be decoded from brain activity in modality-specific cortical areas.

This work is in collaboration with Dr. Thomas Ethofer (Tuebingen), Prof. Klaus Scherer (CISA, Geneva) and Prof. Patrik Vuilleumier (UniGE).

WSPM: Wavelet-Based Statistical Parametric Mapping

We introduce an integrated framework that combines wavelet-based processing with statistical testing in the spatial domain. This approach allow high-spatial resolution brain mapping without the need for spatial smoothing and with proper strong type I error control. The full approach is available as a toolbox, named WSPM, for the SPM2/SPM5 software; it takes advantage of multiple options and features of SPM such as the general linear model.

The associated software can be downloaded here.

BSLIM: Spectral Localization by Imaging with Explicit B0-Field Inhomogeneity Compensation

Magnetic resonance spectroscopy imaging (MRSI) is an attractive tool for medical imaging. However, its practical use is often limited by the intrinsic low spatial resolution and long acquisition time. Spectral localization by imaging (SLIM) has been proposed as a non-Fourier reconstruction algorithm that incorporates spatial a priori information about spectroscopically uniform compartments. Unfortunately, the influence of the magnetic field inhomogeneity—in particular, the susceptibility effects at tissues' boundaries—undermines the validity of the compartmental model. Therefore, we propose BSLIM as an extension of SLIM with field inhomogeneity compensation. A B₀-field inhomogeneity map, which can be acquired rapidly and at high resolution, is used by the new algorithm as additional a priori information. We show that the proposed method is distinct from the generalized SLIM (GSLIM) framework. Experimental results of a two-compartment phantom demonstrate the feasibility of the method and the importance of inhomogeneity compensation.

This work is in collaboration with Dr. Ildar Khalidov (EPFL), Dr. Francois Lazeyras (HUG, Geneva) and Prof. Mathews Jacob (University of Rochester).

Extended Depth-of-Field

Due to the limited depth of field of brightfield microscopes, it is usually impossible to image thick specimens entirely in focus. By optically sectioning the specimen, the in-focus information at the specimen's surface can be acquired over a range of images. Commonly based on a high-pass criterion, extended-depth-of-field methods aim at combining the in-focus information from these images into a single image of the texture on the specimen's surface.
We have first investigated fast methods based on the wavelet that do not rely on an explicit image formation model. For more quantitative evaluation, we propose a method that jointly estimates the texture and topography of a specimen from a series of brightfield optical sections; it is based on an image formation model that is described by the convolution of a thick specimen model with the microscope's point spread function. The problem is stated as a least-squares minimization where the texture and topography are updated alternately. This method also acts as a deconvolution when the in-focus PSF has a blurring effect, or when the true in-focus position falls in between two optical sections.

This work is in collaboration with Francois Aguet (EPFL, BIG).

The associated software can be downloaded here.

Box-Splines for Body-Centered Cubic Lattice

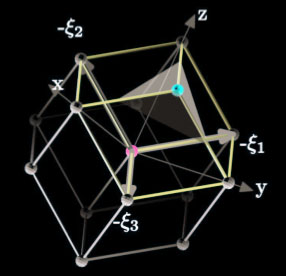We introduce a family of box splines for efficient, accurate, and smooth reconstruction of volumetric data sampled on the body-centered cubic (BCC) lattice, which is the favorable volumetric sampling pattern due to its optimal spectral sphere packing property. First, we construct a box spline based on the four principal directions of the BCC lattice that allows for a linear C0 reconstruction. Then, the design is extended for higher degrees of continuity. We derive the explicit piecewise polynomial representations of the C0 and C2 box splines that are useful for practical reconstruction applications. We further demonstrate that approximation in the shift-invariant space—generated by BCC-lattice shifts of these box splines is twice as efficient as using the tensor-product B-spline solutions on the Cartesian lattice (with comparable smoothness and approximation order and with the same sampling density). Practical evidence is provided demonstrating that the BCC lattice not only is generally a more accurate sampling pattern, but also allows for extremely efficient reconstructions that outperform tensor-product Cartesian reconstructions.

This work is in collaboration with Prof. Torsten Moeller (SFU, Vancouver) and Dr. Alireza Entezari (UFlorida, FL).

Hex-Splines

We have proposed a new family of bivariate, non-separable splines, called hex-splines, especially designed for hexagonal lattices. The starting point of the construction is the indicator function of the Voronoi cell, which is used to define in a natural way the first-order hex-spline. Higher order hex-splines are obtained by successive convolutions. A mathematical analysis of this new bivariate spline family is presented. In particular, we derive a closed form for a hex-spline of arbitrary order. We also discuss important properties, such as their Fourier transform and the fact they form a Riesz basis. We also highlight the approximation order. For conventional rectangular lattices, hex-splines revert to classical separable tensor-product B-splines. Finally, some prototypical applications and experimental results demonstrate the usefulness of hex-splines for handling hexagonally sampled data.
High-quality results for sampling to/from hexagonal lattices can be obtained using quasi-interpolating techniques.

This work is in collaboration with Dr. Laurent Condat (ENSI, Caen).

The associated software can be downloaded here.

Fuzzy-Logic-Based Deinterlacing

We propose a novel algorithm to convert video from the interlaced scanning format to the progressive format. It uses a robust fuzzy logic-based motion detector. Parts of the picture fields with motion are interpolated differently from static parts, in order to obtain excellent results. The algorithm was prototyped into a single FPGA by BarcoView and later used in products such as the MIVD-1218. The prototype device received the "European Information Society Technologies (IST) Prize Nominee" label for 2003.
By clicking on the thumbnail image, one can see a demonstration of the results of fuzzy logic de-interlacer. An original test sequence was interlaced and de-interlaced using different methods, so one can compare the result against the original test sequence. The methods at the top right (line-averaging or "bob") and at the bottom left (field insertion or "weave") are very popular in the 'PC world'. The result at the bottom right was obtained using the fuzzy logic-based approach.